
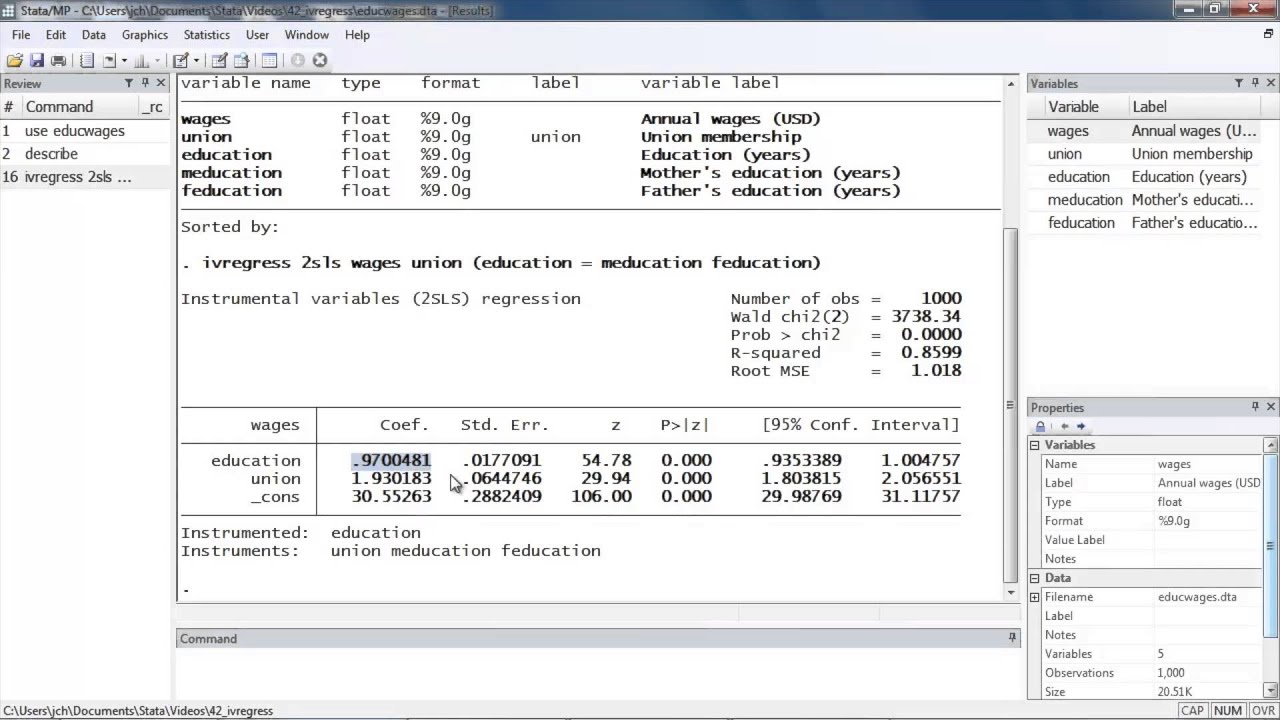
Really, we need to consider at least one threshold point. Looking more closely, the period before the 8th seems to have larger amplitude (height of the wave) than after the 8th. If you compare this to the line chart for temperatures, the size of the sine wave looks fairly sensible, but it is centered around 17 degrees, when actually the month started with temperatures notably above that, which then dropped around the 8th of August to be below that. The intercept term can be interpreted as the average temperature at 11 a.m. This change is 3.2 degrees Celsius, with 95% confidence interval from 2.8 to 3.6. Bear in mind that hoursine varies from -1 to 1, so the coefficient tells us the average change from 5 a.m. If we regress temperature on hoursine, we can evaluate the size of the diurnal variation.
#STATA REGRESS COMMAND SERIES#
We are imposing a sinusoidal shape on this oscillation, and fixing the time of the minimum and maximum, which is not a great idea for time series analysis, but will simplify what follows and allow us to focus on the threshold command. and maximum about 5 p.m., fairly standard in an English summer. Next, we make two new variables: decimalday will be handy for plotting, and hoursine is a quick and dirty way of incorporating the daily oscillation in temperature, with minimum about 5 a.m.

We will open the file (I suggest you browse it to see what's inside) and declare it to be a time series. Let's look at a fairly small dataset: a few weather variables from an observation station to the South of London in August 2018. Identifying the threshold and fitting different models on either side allows you to improve causal understanding or prediction. The post-1986 data would throw your analysis out the birds near human habitation were no longer totally dependent on wild plants. Prior to that point, you might have studied the effect of the size of field margins on farms on the goldfinch population, on the basis that the birds eat seeds of wild plants that grow on the margins of cultivated land.
#STATA REGRESS COMMAND HOW TO#
This is generally ascribed to the birds learning how to forage in suburban gardens. Image copyright British Trust for Ornithology In these population data from the United Kingdom, you can see a sudden change in the time series at around 1986. The goldfinch is a small songbird found throughout Eurasia. The new threshold command allows you to look for these changes in a statistically informed way, which helps you avoid the potential for bias if you just eyeball line charts and pick the point that fits with your expectations.

In time series analysis, sometimes we are suspicious that relationships among variables might change at some time. Stata Tips #17 – Threshold regression for time series in Stata Threshold regression for time series in Stata


 0 kommentar(er)
0 kommentar(er)
